Modified Dietz

Modified Dietz refers to the measure which is used in order to determine the historical performance of the portfolio by dividing the actual cash flow net of the outflow with average capital, which uses the weight and value of the portfolio at the beginning. It is considered one of the most accurate forms of determining the returns from a portfolio.

In a simple Dietz method, all the cash flows are assumed to be from the middle of the period, whereas that is not the case with the modified Dietz method. The latter excludes the external factors which could skew the results of the portfolio. The cash flow mentioned here could refer to withdrawals, fees, or contributions.

• The modified Dietz method is a calculation technique used to determine the rate of return on an investment portfolio that experienced cash flows, such as contributions or withdrawals, during a specific period.
• This method is designed to accommodate portfolios with irregular cash flow patterns, as it considers the timing and magnitude of cash flows in the calculation.
• By incorporating the starting and ending portfolio values and the weighted cash flows, the modified Dietz formula provides a comprehensive measure of portfolio performance for the given period.

Modified Dietz is a method to measure a portfolio’s return historically which is derived from the weighted average of its cashflow. The method assumes that there are regular returns from the portfolio over a specific period and accounts for the timing of the cash flow for its calculation.

The modified Dietz returns are considered more accurate than its counterpart, the simple Dietz method which assumes that the cash flows within a portfolio come from the middle of the evaluation period. This method is named after Peter O Dietz, whose work during the 1960s in relation to calculating the returns of a pension fund investment took prominence and was modified into this method that we widely use today.

As the regulations around the financial sector grow, investors need to take more care regarding how the investment and returns are calculated and how they are reported. This method of Modified Dietz provides reasonable confidence in the investment returns analysis.

The modified Dietz method just provides us with a measure of returns on investment portfolios, where there are multiple inflows and outflows. In the current day, with advanced computing and continuous returns management, this method is not useful. However, the basic concept behind the method is useful for understanding how returns and their calculations work.

The results derived from using this method are also referred to as the modified internal rate of return. However, it is vital to note that this method might lose its accuracy in periods of high volatility in the market of multiple cash flows within a specific period.  

Let us understand the formula to determine the modified Dietz method. This shall act as a basis for our understanding of the calculations and its related factors that shall be discussed further in this article.

• ROR (Rate of Return) – this is the term we are looking to calculate.
• EMV (Ending Market Value) – This is the value of the portfolio after the end of the term we are looking for.
• BMV (Beginning Market Value) – This is the value of the portfolio from the date, which the returns are to be calculated.
• W (Weight of each cash flow on the portfolio) – This is the weight of the portfolio between zero and one, but only between the period they occurred and at the end of the period. This can be explained as the proportion of time between the point in time when the flow occurs and the end of the period. This can be calculated using the formula.
• W = / C, where D is the number of days from the start of the return period until the day on which the flow occurred.
• C (Cash flows during the period) – This might not be a single number, but a series of cash flows that happened during the period.
• W*C = the sum of each cash flow multiplied by its weight. This is a summation of weighted cash flows.

Now that we have a basic understanding of how modified Dietz returns are derived and an outline of its related factors, let us apply the theoretical knowledge to practical application. Let us do so with the help of the examples below.

Example #1

Let us consider a very simple scenario with the following conditions:

• We have a portfolio worth 1 million USD at the beginning of the investment period.
• After two years, the portfolio’s worth has grown to 2.3 million USD.
• There was an inflow of 0.5 million USD after one year.

Now, we will calculate how the Modified Dietz method will be used to calculate the returns in this portfolio.

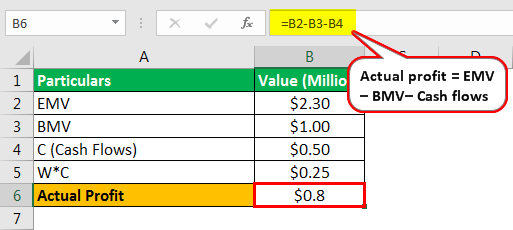
• Actual profit = EMV (2.3 million USD) – BMV (1 million USD) – Cash flows (0.5 million USD Inflow)
• =$0.8

This brings a profit of 0.8 million USD.

Now let us see what the average capital in this case was.

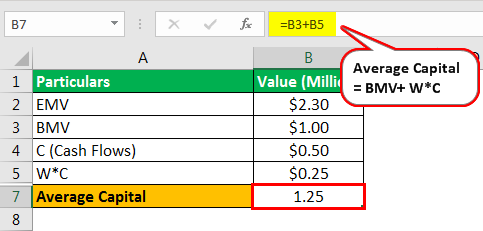
• Average Capital = BMV (1 million USD) + W*C (0.5 million USD * 0.5 Time period)
• = 1.25

Therefore the rate of return will be -

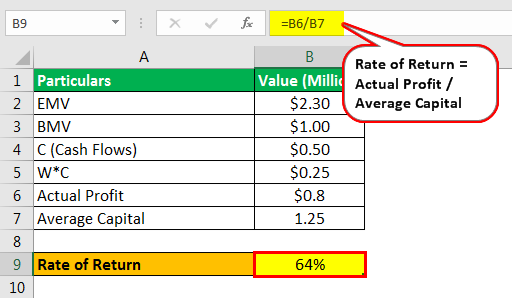
• Rate of Return = Actual Profit / Average Capital
• = $0.8 / 1.25
• = 64%

Example #2

Comparison of Modified Dietz to Time-Weighted Rate of Return

Let us consider two investors with the following portfolios.

1. Investor A started with a portfolio of 250k USD at the beginning of a year (Jan) and used his strategies to make it into 298k USD by the end of the same year (Dec). However, he put up an additional capital of 25k USD in September.
2. Investor B started with a portfolio of 250k USD at the beginning of the year (Jan) and used his strategies but ended up with 251k USD at the end of the year. However, he withdrew 25K in September.

To the naked eye, or by using elementary mathematics in our minds, we can tell that Investor B is worse at investing than Investor A. However, going deep into the calculations will give us another side of the story entirely.

For Investor A:

Actual Profit will be -

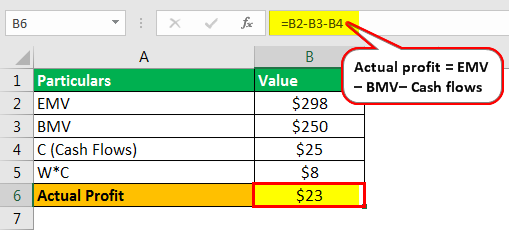
• Actual Profit= (298k USD – 250k USD – 25k USD )
• =23K USD

The average period will be -

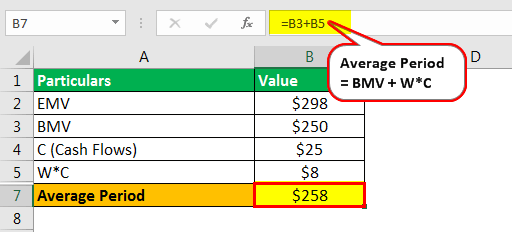
• Average Period = 250k USD + (25k USD * 0.3)
• =258K USD

Modified Dietz Rate will be -

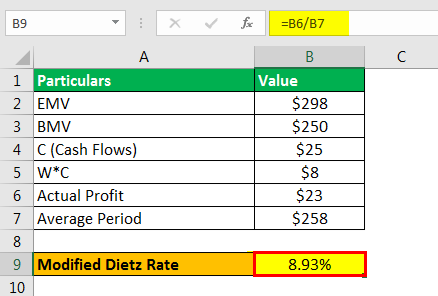
• Modified Dietz Rate = 8.7%

For Investor B:

Actual Profit will be -

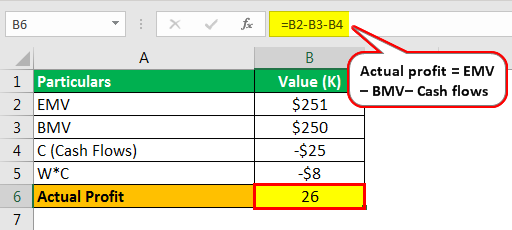
• Actual Profit = (251k USD – 250k USD + 25k USD )
• =26K USD

The average period will be -

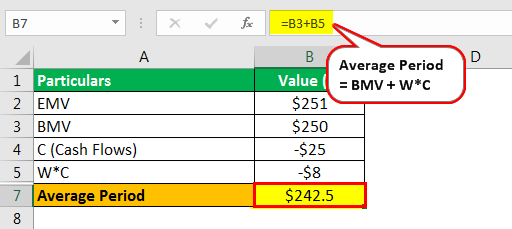
• Average Period = 250k USD + (-25K USD * 0.3)
• =242.5 k USD

Modified Dietz Rate will be -

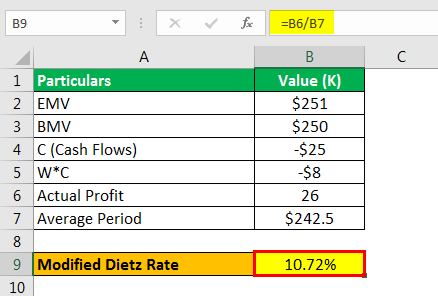
• Modified Dietz Rate = 10.72%

The time-weighted rate of return for both the above will be around 9.5, but modified Dietz gave us different results. This is the reason this method is used by investors for reporting purposes.

Let us understand the advantages of using the modified Dietz method through the points below.

• The main advantage of this method is that it does not require portfolio valuation on each date of the cash flow. This helps the analyst in asserting the value of returns easily, without reassessing each time.
• There are performance attributions that are unavailable with other time-weighing methods; during those cases, the Modified Dietz method is useful.
• Cases like Example 2, where the Time-Weighted Rate of Returns is not an appropriate measure.

Despite the advantages mentioned above, there are a few factors that prove to be a hassle for portfolio managers and investors. Let us understand the disadvantages of modified Dietz returns through the discussion below.

• With the advance in computing, most of today’s returns are calculated on a continuous basis – these provide a better way of analyzing the returns and leave methods like Modified Dietz very naïve and basic.
• An assumption of all transactions taking place simultaneously at a single point in a period of time will lead to errors.
• It is very difficult to deal with negative or average-zero cash flows.

This has been a guide to what is Modified Dietz. Here we explain its formula, examples, advantages, and disadvantages in detail. You can learn more about finance from the following articles -

• Modified Duration Calculation
• Formula of Portfolio Variance
• M2 Measure
• Standard Deviation of a Portfolio
• Financial Planning Apps Softwares